Cosmology is the branch of physics that studies the universe in its largest scales. Specifically, cosmology is mainly concerned with studying the origin, evolution, large-scale structure, dynamics, and eventual fate of the universe. Modern cosmological models are based on the idea that the universe “looks the same”, no matter where we are looking from. This idea is called the cosmological principle.
Of course, such an idea makes no sense on small scales. It is enough to look at our own solar system: a star surrounded by big regions of empty space and an eventual lonely planet. However, if we go to bigger scales, we will see how the differences in local densities begin to average until the idea doesn’t look so crazy anymore.

This view of nearly 10,000 galaxies is called the Hubble Ultra Deep Field. The snapshot includes galaxies of various ages, sizes, shapes, and colours. This is an example of how, when we go to large scales, the universe begins to look homogeneous.
The validity of the cosmological principle is most evident in the observations of the anisotropies of the Cosmic Microwave Background (CMB) carried out by the WMAP and Planck satellites. We’ll talk about the CMB in detail in another article, but for now, it is enough to know that the CMB is electromagnetic radiation that arrives to us from all directions and it follows an almost perfect blackbody spectrum with a temperature of $3$ Kelvin. It is believed to be a remnant from an early stage of the universe. The CMB is almost perfectly smooth, to the point that variations in its regularity are of the order of $10^{-5}$ or less.
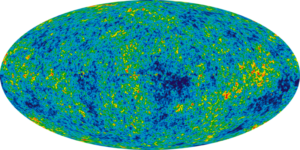
Map of the temperature fluctuations of the CMB (shown as color differences) created from nine years of WMAP satellite data. This image shows a temperature range of ± 200 microKelvin.
The cosmological principle is actually related to two precise properties that a manifold can have: isotropy and homogeneity. The isotropy applies in a particular point of the manifold and basically means that the manifold looks the same no matter in what direction you look. The observations of the CMB mentioned before provide experimental evidence that the universe is isotropic.
The other property we mentioned is homogeneity, if a manifold is homogenous, it means that the metric is the same throughout the manifold. A manifold can be homogeneous without being isotropic around any point, or it can be isotropic around a point without being homogeneous. However, if a manifold is isotropic everywhere, then it is homogeneous. Of course, these two properties have a rigorous mathematical definition, which we will study another time.
Since the CMB seems to imply that the universe is isotropic, and the Copernican Principle tells us that we are not privileged observers in the universe, then other observers in other regions of the universe should also observe this isotropy. Therefore, we can assume that the universe is both homogeneous and isotropic. This is exactly what the cosmological principle states.
At this point, we can be tempted to assume that spacetime is homogeneous and isotropic. However, evidence from observations of type II-A supernovas tells us that the universe evolves in time and is currently undergoing an accelerated expansion. The conclusion should then be that the universe is homogeneous and isotropic in space but not in time.
Then, if we want to use the ideas of General Relativity to describe our universe, we need to look for a metric that obeys the conditions specified above: the universe is homogeneous and isotropic in space but evolves in time. Such a metric is called the Friedmann-Lemaître-Robertson-Walker metric and we will describe it in the next article.
